As we already had a brief insight from one of our previous articles, 'Stability' is defined as the phenomenon of a ship to resist external or internal loads on it and to acquire its original upright state on removal of the external or internal loads. Stability is a crucial phenomenon governing ship design and seakeeping performance of a vessel.
Moreover they can be classified into two types:
· Intact stability
· Damage stability
But how much are we sure of the fact that a vessel becomes unstable only when there is undamaged condition? In fact, the reverse is in often more likely, that is the ship has suffered a breach or damage and is structurally affected. This in turn has triggered flooding of water leading to loss in stability. Loss of stability can also be caused by other factors without involving damage, various causes being explained in the previous article.
 |
| Figure 1: (Copyright: Wordpress) |
DAMAGE STABILITY
This type of stability concerns with stability of the ship when it is damaged, usually hull is breached. It includes of flooding of ship compartments when hull is damaged leading to sinkage of the ship below margin line or even total sinkage or capsize of the ship. In this article we would focus mainly on damage stability.
A ship gets damage or suffers a breach mainly because of one of the following causes or sometimes their combination:
- Collision: This is a very common reason often leading to adverse effects. Collision may be with another vessel (remember Titanic?) or some landmass like harbor, port, reef or island. Most of them are caused by compounded operator error, carelessness, technical flaws, machinery and equipment failure, problems in maneuverability, accidents or sometimes unavoidable circumstances leading to damage.
- Grounding: Grounding is often caused by improper draft considerations in water bodies, excessive trim or in shallow draft conditions.
- Structural problems: Sometimes there is lack of structural soundness due to manufacturing defects, improper behaviour, lack of maintenance, fatigue or unprecedented loading.
- Environmental Vagaries like rogue waves, cyclones, sea-storms, heavy rainfall or sometimes cold weather conditions leading to ice accretions. It may be worthwhile to mention that icebergs which are very much prevalent in northern seas are very big problems for navigation which can often lead to precarious collisions just like in case of Titanic.
CLASSIFYING DAMAGE STABILITY
So far we have introspected upon the causes that hampers the stability of the vessel after wreaking damage. But broadly, damage stability may be classified into two groups:
- Deterministic Damage Stability
- Probabilistic Damage Stability
Deterministic Damage Stability
This is the traditional old-school technique for assessing stability of the ship when it is flooded. In this process the ship is divided into several subdivisions along its length with the help of transverse watertight bulkheads. Now the stability of the ship is calculated when one or more compartments gets flooded due to breach of hull.
This is the most common problem in regard to stability. However, it is treated differently in case of intact and damage stability.
In intact stability, the preliminary condition is that the ship remains 'undamaged'. Now what could be the cause for a vessel to capsize without suffering physical damage? The answer is simple. As the loss of stability is solely caused by the loss of equilibrium of forces, the most occurrent cause is due to shifting of cargo or injudicious distribution of weights (cargo, ballast, machinery etc.) which triggers of the ship to heel to one side in a local phenomenon termed as Listing. However, in dealing with damage stability problems, we isolate the causes caused due to internal effects and merely concentrate on the damaged aftermath stability conditions.
In damage stability criterion, capsizing is caused due to the breaching of hull after suffering damage sideways which can cause water flooding in that region only (tanks, cargo spaces etc.). This sudden flooding of water causes the ship to heel to one side accounting to its loss in equilibrium.
As illustrated in the figure, unwanted flooding of a compartment or space leads to drastically altered buoyancy forces along with their lines of action. This difference in the line of action of the overall buoyancy of the damaged ship with respect to the weight still acting through its center of gravity creates a large heeling moment causing it to topple and finally capsize. As a result, it heels over large angle such that its righting moment is insufficient and it topples over. This happened in the disaster of ship COSTA CONCORDIA due to the collision.
WAYS IN WHICH A SHIP SINKS
A ship can sink in usually 3 ways when its hull is damaged giving way for flooding.
FOUNDERING
It is the case when a ship runs over some reef or rock and it damages its bottom keel and consequent flooding occurs. Due to flooding the ship’s draft increases to compensate the lost buoyancy and sinks when Weight > Buoyancy. One important thing is that foundering does not necessary lead to heeling of ship is the weight distribution is still properly maintained. However, due to flooding of the damaged compartments may lead to direct sinkage and sometimes trim.
Figure 2: Damage in hull due to Foundering (Courtesy: NEEC)
CAPSIZING
In intact stability, the preliminary condition is that the ship remains 'undamaged'. Now what could be the cause for a vessel to capsize without suffering physical damage? The answer is simple. As the loss of stability is solely caused by the loss of equilibrium of forces, the most occurrent cause is due to shifting of cargo or injudicious distribution of weights (cargo, ballast, machinery etc.) which triggers of the ship to heel to one side in a local phenomenon termed as Listing. However, in dealing with damage stability problems, we isolate the causes caused due to internal effects and merely concentrate on the damaged aftermath stability conditions.
In damage stability criterion, capsizing is caused due to the breaching of hull after suffering damage sideways which can cause water flooding in that region only (tanks, cargo spaces etc.). This sudden flooding of water causes the ship to heel to one side accounting to its loss in equilibrium.
As illustrated in the figure, unwanted flooding of a compartment or space leads to drastically altered buoyancy forces along with their lines of action. This difference in the line of action of the overall buoyancy of the damaged ship with respect to the weight still acting through its center of gravity creates a large heeling moment causing it to topple and finally capsize. As a result, it heels over large angle such that its righting moment is insufficient and it topples over. This happened in the disaster of ship COSTA CONCORDIA due to the collision.
 |
| Figure 3: The capsizing of ship due to heeling moment |
PLUNGING
This depicts damage on a longitudinal basis. This aspect of damage generally deals with flooding in the fore and act regions. The causes can be in plenty from head-on collisions (like in a case of Titanic) to leakage in the hull skin. The unwanted seepage of water in the fore and aft is not as severe as capsizing. Moreover, with the development of watertight bulkheads dividing the hull into numerous watertight compartments from fore to aft, the risk is less posed (as even if the fore or the aft peak bulkhead got flooded, the remaining watertight compartments would remain safe due to their watertight integrity). Plunging only has a negative impact of trim (either by bow or stern). Trim is much more acceptable as compared to sideways heel when it comes to stability criterion but still can have detrimental consequences if deck immersion takes place, that is waterline reaches up to the weather deck and then flooding it. In the worst case scenarios, this creates a high trimming moment beyond revival making the ship succumb to its damage, sinking it by bow or stern.
The following figures illustrate.
Figure 4: Gradual Capsizing due to trim
EFFECT OF FLOODING ON STABILITY
We have so far expressed concerns about flooding which can affect stability. But have we thought about the simple physics that governs them?
When a ship floods its buoyancy is lost but displacement V remains same. So the draft increases to regain the lost buoyancy. As the draft increases the waterplane area (AW) decreases. Thus
View = Vold , the volume remaining the same
Aw(new)<Aw(old), accounting to the hull form
So,
So,
IT(new) < IT(old) , Area Moment of inertia of waterplane
We know, from stability calculation relations BM= IT /V .
So BMnew < BMold .
GM = KB + BM – KG .
Figure 5 : Courtesy: NEEC
Now due to flooding KB will increase (due to increased draught) and BM will decrease (see figure). As a combined result GM decreases. Now as GM decreases the righting lever GZ decreases and the stability of the ship as a whole,decreases (refer figure 5).
 |
| Figure 6: Conditions of Transverse Statical Stability |
SUBDIVISION AND FLOODABLE LENGTH
In deterministic approach of damage stability, the ship is divided into several subdivisions. This is done to restrict flooding of the ship on hull damage.
 |
| Figure 7: Division into watertight bulkheads (Image Courtesy: NEEC) |
Essentially in all ships, the hull is divided into a number of watertight compartments by the means of watertight bulkheads. The physics behind this development is intrinsically related to damage stability. Say, on a particular occasion, the ship hull gets breached. It will get flooded, of course. But with the subdivision into compartments, the risk of sinking is very much lowered. Even if flooding occurs, it is limited to one or a few bulkheads. On the contrary, if there were no bulkheads, the entire hull (Cargo holds, machinery spaces, engine room, accommodation etc) would have flooded beyond limits, making the ship the to sinkage.However, if damage takes place across numerous watertight compartments beyond the maximum limits of flooding (change in draft, of course), the ship may be in the precarious conditions of sinkage.Remember Titanic? It had grazed past the iceberg in an attempt to maneuver the past, inflicting damage to many of its compartments comprising for its watertightness, resulting in its deadly aftermath. Instead, if it would have directly rammed into the iceberg, the Fore peak tank and at most a couple of subsequent compartments would have flooded saving the rest. This could have prevented the catastrophe.
Thus, invariably all modern designs maximize the number of watertight bulkheads to account for 'Damaged Stability.'
Here all subdivisions are done according to its ability to resist flooding in damaged conditions to the safest limits. Floodable Length is an important parameter taken into account here. It is defined as the maximum length of the compartment that can be flooded such that the draft of the ship remains below the margin line. Thus, maximum division of bulkheads is the best solution. But, other factors such as minumum required size of hold, improper cargo stowage, more number of required outfittings or increased steel weight hinders the possibility to some extent. Thus, optimizing the safe limits of floodable length to the minimum required length of watertight compartment are done in most cases.
 |
| Figure 8: Watertight Subdivision of a ship (Courtesy: Wikipedia) |
FLOODABLE LENGTH CURVE
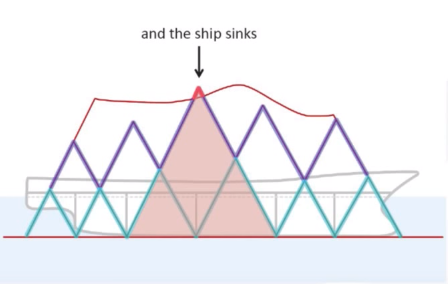
Suppose the ship is divided into a certain number of transverse bulkheads. Now each compartment has its floodable length. The lengths of the floodable lengths are plotted vertically from the midpoints of the horizontal floodable lengths and these points are joined together.
 |
| Figure 9: Floodable Lengths (Image Courtesy: NEEC)
The end and starting points of the curve is joined with the aft and forward keel of the ship (profile view). This gives us the floodable length curve.
Figure 10: Floodable Length curve (Image Courtesy:NEEC)
|
At first for a vessel the factor of subdivision (FOS) is calculated. The FOS depends on the length of the ship and other numerical factors. The inverse of FOS gives the compartment standard of the ship. One compartment standard means the ship will survive if one compartment is flooded. The product of FOS and floodable length gives the permissible length. The length of a compartment cannot be greater than permissible length. The area under floodable length curve is the maximum extent a ship can be allowed flooding to prevent the ship to sink. For a single compartment, flooding triangles are formed from the edges of the bulkheads with the height equal to permissible length. This is the actual floodable length curve for single compartment flooding. This curve also gives the stability of the ship if one or multiple compartments are damaged.
 |
| Figure 12: Image Courtesy:NEEC |
If for any single or group of compartments flooding the area of the actual floodable length curve exceeds the allowable floodable length curve then the ship will sink.
Thus if the third bulkhead from aft of the ship damages the ship will sink since it’s floodable length curve exceeds the allowable floodable length curve.
Figure 11: Floodable Length estimates for a damaged hull profile (Image Courtesy: NEEC)
PROBABILISTIC DAMAGE STABILITY
In deterministic approach we had subdivision to restrict flooding and the sinkage of the ship if one or more compartments are flooded can be retrieved from floodable length curve. But in reality, we don’t know whether a ship will be damaged in a voyage or not. If it’s damaged how many compartments will be flooded? Will it be damaged by aft or forward? Will it plunge or capsize or founder? There is a lot of uncertainty involved.
The theory of probability solves this problem. With the help of probability, we find,
- How ships are damaged?
- How often is a part damaged?
- What is the chance of survival if that part of ship is damaged?
We find the probability of each case and multiply the probabilities of each constraint. Finally, we add the total probability to get the chance of survival of the ship according to the damage occurred.
Figure 12: Sample Probabilistic Tables
ATTAINED SUBDIVISION INDEX
 |
| Figure 13 |
Attained subdivision index is the sum of all probability of surviving for 1 compartment flooding, 2 compartment flooding, etc.
A = P(one compartment flooding) + P(2 compartment flooding) + etc.
A > R.
Where R = the required subdivision index.
Thus the damaged stability of vessels is calculated which decides the overall stability of the ship when it is damaged and tell whether the ship will survive sustaining the respective damage.LSD
Article By: Rijay Majee
